Polyhedral Cells in Natural Structures
Cellular structures are found in many natural systems, including biological tissue, soap foams, and polycrystalline metals. The building blocks of these three-dimensional structures are three-dimensional polyhedra. In many situations, these cells are topologically equivalent to simple, convex polyhedra. Although these polyhedra can be enumerated (see here for instance), a few questions naturally arise. In a given structure, do certain types of cells appear more frequently than other types? If so, which types occur more or less frequently and why?
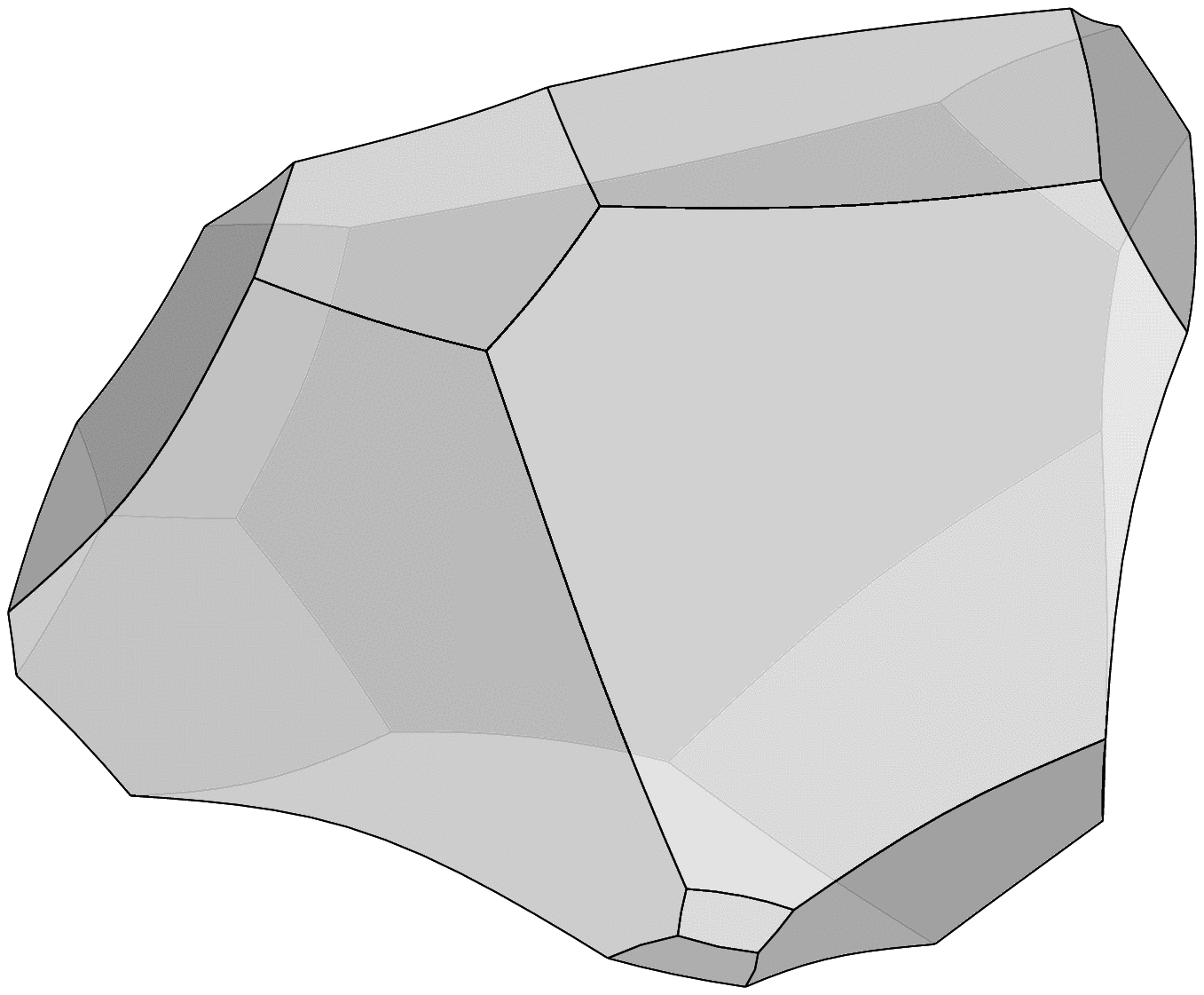
For at least two natural structures, the answer to the first question is an emphatic yes; see here and here, which describe the types of polyhedral cells that arise in Poisson-Voronoi and grain-growth structures. In both cases we observe a strong preference for certain types of cells. Answers to the second questions are the subject of current work.
- Lazar, E.A., Mason, J.K., MacPherson, R.D. and Srolovitz, D.J. "Complete topology of cells, grains, and bubbles in three-dimensional microstructures", arXiv, Phys. Rev. Lett. 109:095505, 2012.
- Lazar, E.A., Mason, J.K., MacPherson, R.D. and Srolovitz, D.J. "Statistical topology of
three-dimensional Poisson-Voronoi cells and cell boundary networks", arXiv, Phys. Rev. E. 88:063309, 2013.
Return to other research interests.
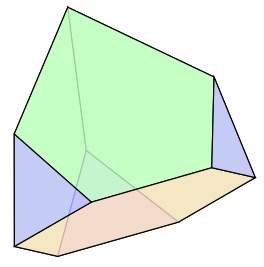
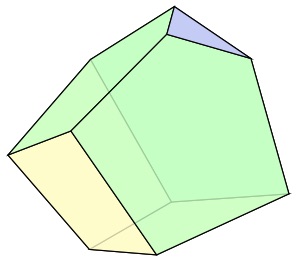
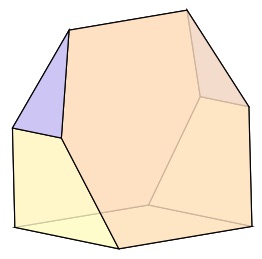
All possible simple, convex polyhedra with 7 faces;
each polyhedron is drawn in 'canonical form'.