Summary
My research interests are divided between two primary areas: topological methods of characterizing the structure of large cellular and atomistic data sets, and the short- and long-term evolution of dynamical cell structures. Both areas have important applications in understanding the physics of materials.Topological Methods in Structure Analysis
Many physical problems involve understanding how a set of points are arranged in space. Continuous, real-valued order parameters are
frequently used to described local structure in such data sets. Despite their success in analyzing many physical systems, such
methods also have certain limitations. Topological methods provide an alternate way to think about local structure which avoids some limitations of continuous methods, and enable robust tools for practical computations.
Grain Growth
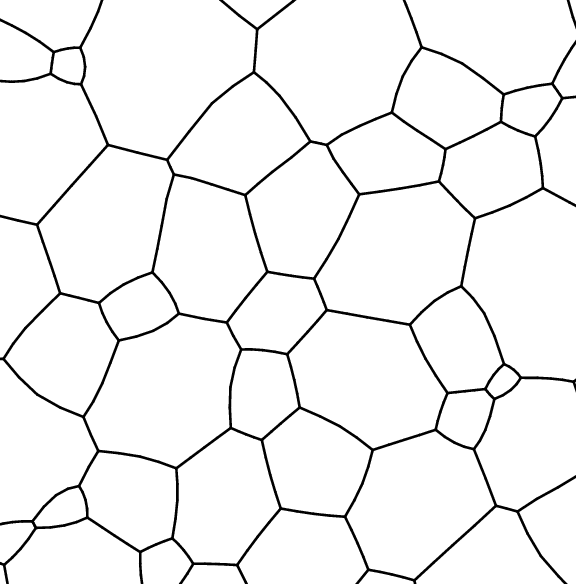Many cellular structures that appear in nature -- including biological tissue, soap foams, polycrystalline metals, and international borders -- evolve over time in complicated ways. Understanding how these systems evolve is crucial to understanding large-scale properties of these systems. Click here to learn more.
Polyhedral Cells in Natural Structures
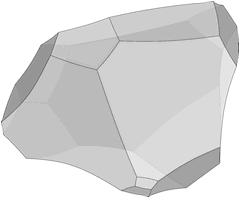I am interested in understanding the (roughly) convex polyhedra that are found in disordered cellular structures. Among other properties, we consider the frequency with which we find certain topological types in various structures. Click here to learn more.
Three-Dimensional Poisson-Voronoi Tessellations
Voronoi tessellations of Poisson point processes are widely used for modeling many types of physical and biological systems. Here we analyze simulated Poisson-Voronoi structures containing a total of 250,000,000 cells to provide topological and geometrical statistics of this important class of networks.Schlegel Diagrams
A Schlegel diagram is the projection of a polytope from d-dimensional space into d-1 dimensions. Schlegel diagrams can be useful for studying three-dimensional polyhedra. Click here for a brief explanation.
Mechanical Properties of Metal Fracture
During my last year in college and in the summers following, I studied how polycrystalline materials crystallize and fracture. Using computers to simulate metals, we looked at how grains form and how the size and orientation of the grains impact mechanical properties of materials such as strength and elasticity.This project developed molecular dynamics methods to cool samples, apply strains, measure stresses, and calculate relevant properties such as grain size and orientation. We outlined the challenge of grain identification and its inherent difficulties, and developed algorithms to computationally identify grains and grain boundaries in systems of particles. We reproduced experimental data illustrating the influence of cooling rates on average grain sizes and data associating higher ultimate tensile strength with larger grains at the nanometer level. We also investigated the relationships between average grain size and Young's modulus of elasticity, showing that Young's modulus increases, perhaps linearly, with grain size; the relationship between grain orientation and ultimate tensile strength, demonstrating that certain orientations are associated with higher ultimate tensile strength; and the relationship between grain orientation and Young's modulus of elasticity (we saw no correlation between these properties).
This work was guided by Shlomo Ta'asan, of the Center for Nonlinear Analysis at Carnegie Mellon University. Click here for a copy of my thesis on this subject. Comments are always welcome.